Fingertip Reaction Time
An educational, fair use website
Abstract
The purpose of this experiment is to compare the fingertip reaction times of male and female students to male and female teachers of Midwood High School.
Introduction
Reaction time is the time discrepancy between the moment of change in the environment and the beginning of your response. Fingertip reaction time is tested by dropping a ruler between the outstretched fingers of the subject without warning. The distance fallen can be used to determine the time to react to the event.
You might ask why some people just have a higher reaction time than others. First, you must understand how you, as a person, reacts to a change in the environment. For instance, when the subject saw the experimenter drop the ruler, it took some time for the brain to realize that the ruler was being dropped. (That's why you need a friend, you can't just drop the ruler yourself and catch it and assume that you have such a high reaction time!)
Generally speaking, your nervous system is divided into two parts with a central nervous system (consisting of the brain and the spinal cord) and a peripheral nervous system (composed of all the nerves that deliver messages to the spinal cord). Both parts are at work here. First, the nervous system must recognize a stimulus (the ruler being dropped), then cells in the nervous system called neurons relay the message to the brain, muscles and other nerves. Now the peripheral nerve comes into play: the message travels from the brain to the spinal cord and is finally delivered to your fingers. The motor neurons tell the muscles to catch the ruler.
For more information, visit:
Using the formula
s = so + vot + ½at2
where s is the distance, vo is the initial velocity, t is the time, and a is the acceleration, your reaction time can be measured given the distance you catch the ruler at and that you release the ruler from rest. Many believe that males have a quicker reaction time overall than females. This experiment is to confirm whether or not this claim is true. The average reaction time of a human is approximately between 0.2 s to 0.25 s. However, your reaction time is also affected by factors such as age, gender, intelligence, fatigue, and distraction.
Procedure
- Randomly pick a male or female student to perform this simple experiment.
- Ask them to extend their hand and hold out their thumb and forefinger.
- The 0 centimeter mark on the ruler should be level in between the subject's fingers.
- Ask them to catch the ruler with these two fingers. Do not give any advanced notice when you release it from rest.
- Record the position of their fingers on the ruler when they catch it.
- Repeat the first 5 steps for more trials.
- Repeat the first 6 steps with teachers (male and female) as your test subject.
Analysis
Testing you reaction time is as simple as 123. With a ruler and a friend, you can tell how long a person it takes to react to a change. Having a fast reaction time is vital in sports whether you're in the track, hockey or soccer (especially a goalie) teams, etc.
In our experiment, one person will drop the ruler and when the subject catches it, you can measure the reaction time. First, we convert the centimeters to meters for a standard measurement. Then, using the distance formula given in the introduction
s = ½at2
since there is no initial distance nor initial velocity. So the time can be found from
t = √2s/a
 |
|
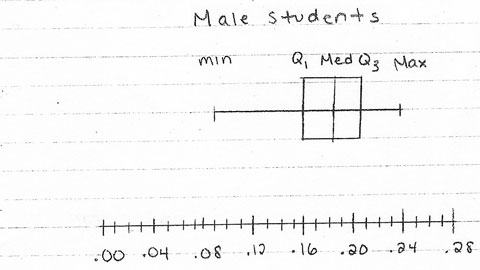
| mean μ = | 0.1778 s |
| standard deviation σ = | 0.0344 s |
| minimum value | 0.0903 s |
| first quartile Q1 = | 0.1596 s |
| median | 0.1806 s |
| third quartile Q3 = | 0.2044 s |
| maximum value | 0.2389 s |
| number of samples | 62 |
| Data Table for Male Students | |
 |
|
| mean μ = | 0.1950 s |
| standard deviation σ = | 0.0338 s |
| minimum value | 0.0553 s |
| first quartile Q1 = | 0.1766 s |
| median | 0.2019 s |
| third quartile Q3 = | 0.2189 s |
| maximum value | 0.2473 s |
| number of samples | 71 |
| Data Table for Female Students | |
 |
|
| mean μ = | 0.2033 s |
| standard deviation s= | 0.0236 s |
| minimum value | 0.1564 s |
| first quartile Q1 = | 0.1848 s |
| median | 0.1968 s |
| third quartile Q3 = | 0.2279 s |
| maximum value | 0.2473 s |
| number of samples | 20 |
| Data Table for Male Teachers | |
 |
|
| mean μ = | 0.1878 s |
| standard deviation s = | 0.0262 s |
| minimum value | 0.1355 s |
| first quartile Q1 = | 0.1713 s |
| median | 0.1915 s |
| third quartile Q3 = | 0.2118 s |
| maximum value | 0.2258 s |
| number of samples | 20 |
| Data Table for Female Teachers | |
Conditions for a 2-Sample T-Interval:
- 10% of the population: There are 3,695 students and 218 teachers in Midwood High School in the spring of 2006. Of the students, 1,667 of them are male and 2,028 are female. Of the teachers, 102 of them are male and 116 of them are female. Since there are countless schools in the United States, we can assume that we did take less than 10% of the population of students and teachers.
- approximate normal distribution: We based our sample on a large population.
Based on a 95% confidence interval test to compare 2 samples, we performed four 2-Sample T-Intervals to find if there was a significant difference in the interval of the true fingertip reaction times. First, we let μo = the average fingertip reaction time. Then, we set our null hypothesis, Ho: μ1 = μ2, and our alternative hypothesis, HA : μ1 = μ2. We find our interval from the formula
(μ1–μ2) ± t*√(s12/n1 + s22/n2)
where s is the standard deviation and n is the number of samples.
- Let μ1 = the mean reaction time of male teachers
- Let μ2 = the mean reaction time of the female teachers
2- Sample T-Interval
(−9 × 10−4, 0.03189) with df = 37.6198
Since 0 is in the interval, we don't reject the null hypothesis. Therefore, there's no difference in the reaction time of male and female teachers.
- Let μ1 = the mean reaction time of male students
- Let μ2 = the mean reaction time of the female students
2- Sample T-Interval
(−0.029, −0.0053) with df = 127. 976
Since 0 is not in the interval, we reject the null hypothesis. Therefore, there's difference in the reaction time of male and female students. Since it's a negative number, males are between 0.0029 and 0.0053 seconds faster than the female students.
- Let μ1 = the mean reaction time of male students
- Let μ2 = the mean reaction time of the male teachers
2- Sample T-Interval
(−0.0397, −0.0115) with df = 45.8719
Since 0 is not in the interval, we reject the null hypothesis. Therefore, there's difference in the reaction time of male students and male teachers. Since it's a negative number, male students are between 0.0397 and 0.0115 seconds faster than the male teachers.
- Let μ1 = the mean reaction time of female students
- Let μ2 = the mean reaction time of the female teachers
2- Sample T-Interval
(−0.0076, 0.03189) with df = 37.6198
Since 0 is in the interval, we don't reject the null hypothesis. Therefore, there's no difference in the reaction time of female students and teachers.
We found that females have the minimum value for reaction time: 0.0553 seconds while the minimum male reaction time value is 0.0903 seconds. However, overall, male students' reaction time was the fastest when we performed a 95% confidence interval. The histogram for male student reaction time is slightly skewed left with no outliers and for female, it's skewed left with one outlier. Surprisingly, we found that there was no difference in the reaction times between male and female teachers when we performed a 95% confidence interval. Both of the histograms are approximately symmetric with no outliers.
Conclusion
According to our statistical analysis of the fingertip reaction time, our experiment showed that male students in Midwood high school have the fastest reaction time. However, Literature Review on a reaction time stated that,
In a surprising finding, Szinnai et al. (2005) found that gradual dehydration (loss of 2.6% of body weight over a 7-day period) caused females to have lengthened choice reaction time, but males to have shortened choice reaction times. Adam et al. (1999) reported that males use a more complex strategy than females. Barral and Debu (2004) found that while men were faster than women at aiming at a target, the women were more accurate.
We also performed an analysis on male students and teachers to find out if reaction time depends on age. We found that there's a significant difference between male students and teachers while there's no considerable difference between female students and teachers.
The average reaction time is 0.1778 s for male students, 0.1950 s for female students, 0.2033 s male teachers, and 0.1878 s for female teachers (refer to the graph for the rest of the information: median, quartile, etc.).
Sources of Error
- One source of error was in that there was a sampling error since we didn't have a specific age group for the teachers. For the students, the age group was specifically from 15 to 18 years of age. However, the teachers' age group ranged from their mid-20s to their 60s.
- Since we didn't take a simple random sample (SRS) of the population, we cannot assume that this conclusion represents the whole population. This conclusion can only be represented as the results for the population of interest (Midwood High School).
- There was also an experimental error since the distance measurement was subjective. We made a estimate by approximating to the middle of the fingers and rounding to the nearest centimeter.
- We only used a ruler that was 12 inches (30 centimeters) long. However, some of the subjects were unable to catch it within those 30 centimeters so we simply repeated the trial again until they could catch it.
Shu Mei Deng, Sahrish Javed, Julie Tan with Natalie Weng -- 2005
Students Choice: